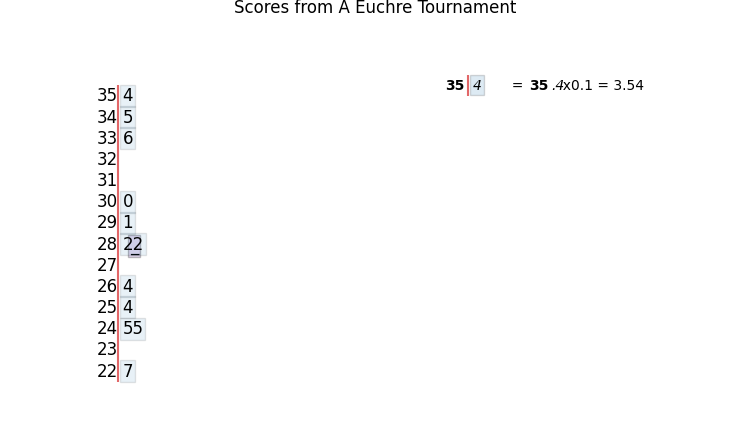
If skill is important to a player’s Euchre score, the skill of the partner and opponents should be important. Therefore, I would expect scores in games with a winning partner to be higher than average and with winning opponents to be lower than average. I define a winning player as one whose average score is above the 95% confidence limits around the overall mean score. In this case a winning player has a mean score of 3.1 or better. As can be seen from the stem-leaf plot, three players meet this criterion.
I computed the average for all scores in games with a winning partner, all scores in games against 1 winning opponent, and all scores in games against 2 winning opponents. I compared the means against the overall mean score by using t-tests with pooled values for the number of measurements and standard deviations.
| Games with a winning partner | Games against 1 winning opponent | Games against 2 winning opponents | |
| Average Score | 3.5 | 2.9 | 1.0 |
| Student’s t | 1.56 | -0.01 | -10.70 |
| Probability of this t-score occurring due to random variation | 0.08 | 0.50 | 0.00 |
As can be seen from the computed t-values and the probabilities of those t-values occurring due to random variation, there was a benefit (at more than a 90% confidence level) to playing with a winning player and a significant disadvantage (at more than a 99% confidence level) to playing against two winning players. Since the presumed lack of skill by a non-winning player lessens the benefit from the presumed skill of a winning player, I find it unsurprising that playing against just one winning opponent had no statistically significant effect. These calculations continue to support my assertion that Euchre is a game of skill. It also seems that the power of a partner or opponent is strong indeed.
All of these calculations have been based on the assumption that the hands each player is dealt end up being statistically similar once the entire tournament is taken into account. Next, I’ll need to figure out if this is a reasonable assumption.


